 As a simple application of the notion of electric flux, let us consider the total flux through a sphere of radius r, which encloses a point charge q at its centre. Divide the sphere into small area elements,
As a simple application of the notion of electric flux, let us consider the total flux through a sphere of radius r, which encloses a point charge q at its centre. Divide the sphere into small area elements, The flux through an area element dS is where we have used Coulomb’s law for the electric field due to a single charge q. The unit vector ˆr is along the radius vector from the center to the area element.Now, since the normal to a sphere at every point is along the radius vector at that point, the area element dS and ˆr have the same direction. Therefore,
The flux through an area element dS is where we have used Coulomb’s law for the electric field due to a single charge q. The unit vector ˆr is along the radius vector from the center to the area element.Now, since the normal to a sphere at every point is along the radius vector at that point, the area element dS and ˆr have the same direction. Therefore,since the magnitude of a unit vector is 1. The total flux through the sphere is obtained by adding up flux through all the different area elements:
Since each area element of the sphere is at the same distance r from the charge,
We state Gauss’s law without proof:
Electric flux through a closed surface S
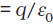
The law implies that the total electric flux through a closed surface is zero if no charge is enclosed by the surface. We can see that explicitly in the simple situation. Here the electric field is uniform and we are considering a closed cylindrical surface, with its axis parallel to the uniform field E. The total
flux f through the surface is f = f1 + f2 + f3, where f1 and f2 represents the flux through the surfaces 1 and 2 (of circular cross-section) of the cylinder and f3 is the flux through the curved cylindrical part of the closed surface. Now the normal to the surface 3 at every point is perpendicular to E, so by definition of flux, f3 = 0. Further, the outward normal to 2 is along E while the outward normal to 1 is opposite to E. Therefore,
where S is the area of circular cross-section. Thus, the total flux is zero, as expected by Gauss’s law. Thus, whenever you find that the net electric flux through a closed surface is zero, we conclude that the total charge contained in the closed surface is zero.
The great significance of Gauss’s law Eq., is that it is true in general, and not only for the simple cases we have considered above. Let us note some important points regarding this law:
(i) Gauss’s law is true for any closed surface, no matter what its shape or size.
(ii) The term q on the right side of Gauss’s law, Eq., includes the sum of all charges enclosed by the surface. The charges may be located anywhere inside the surface.
(iii) In the situation when the surface is so chosen that there are some charges inside and some outside, the electric field [whose flux appears on the left side of Eq. is due to all the charges, both inside and outside S. The term q on the right side of Gauss’s law, however, represents only the total charge inside S.
(iv) The surface that we choose for the application of Gauss’s law is called the Gaussian surface. You may choose any Gaussian surface and apply Gauss’s law. However, take care not to let the Gaussian surface pass through any discrete charge. This is because electric field due to a system of discrete charges is not well defined at the location of any charge. (As you go close to the charge, the field grows without any bound.) However, the Gaussian surface can pass through a continuous charge distribution.
(v) Gauss’s law is often useful towards a much easier calculation of the electrostatic field when the system has some symmetry. This is facilitated by the choice of a suitable Gaussian surface.
(vi) Finally, Gauss’s law is based on the inverse square dependence on distance contained in the Coulomb’s law. Any violation of Gauss’s law will indicate departure from the inverse square law.
1. Applications of Gauss's Law
The electric field due to a general charge distribution is, as seen above, given by equation. In practice, except for some special cases, the summation (or integration) involved in this equation cannot be carried out to give electric field at every point in space. For some symmetric charge configurations, however, it is possible to obtain the electric field in a simple way using the Gauss’s law. This is best understood by some examples.
1.1 Field due to an infinitely long straight uniformly charged wire
Consider an infinitely long thin straight wire with uniform linear charge density l. The wire is obviously an axis of symmetry. Suppose we take the radial vector from O to P and rotate it around the wire. The points P, P¢, P¢¢ so obtained are completely equivalent with respect to the charged wire. This implies that the electric field must have the same magnitude at these points. The direction of electric field at every point must be radial (outward if l > 0, inward if l < 0). Consider a pair of line elements P1 and P2 of the wire, as shown. The electric fields produced by the two elements of the pair when summed give a resultant electric field which is radial (the components normal to the radial
vector cancel). This is true for any such pair and hence the total field at any point P is radial. Finally, since the wire is infinite, electric field does not depend on the position of P along the length of the wire. In short, the electric field is everywhere radial in the plane cutting the wire normally, and its magnitude depends only on the radial distance r. To calculate the field, imagine a cylindrical
Gaussian surface. Since the field is everywhere radial, flux through the two ends of the cylindrical
Gaussian surface is zero. At the cylindrical part of the surface, E is normal to the surface at every point, and its magnitude is constant, since it depends only on r. The surface area of the curved part is 2(pie)rl, where l is the length of the cylinder.
vector cancel). This is true for any such pair and hence the total field at any point P is radial. Finally, since the wire is infinite, electric field does not depend on the position of P along the length of the wire. In short, the electric field is everywhere radial in the plane cutting the wire normally, and its magnitude depends only on the radial distance r. To calculate the field, imagine a cylindrical
Gaussian surface. Since the field is everywhere radial, flux through the two ends of the cylindrical
Gaussian surface is zero. At the cylindrical part of the surface, E is normal to the surface at every point, and its magnitude is constant, since it depends only on r. The surface area of the curved part is 2(pie)rl, where l is the length of the cylinder.
Flux through the Gaussian surface
= flux through the curved cylindrical part of the surface
= E × 2(pie)rl
= flux through the curved cylindrical part of the surface
= E × 2(pie)rl

where ˆn is the radial unit vector in the plane normal to the wire passing through the point. E is directed outward if l is positive and inward if l is negative.
Note that when we write a vector A as a scalar multiplied by a unit vector, i.e., as A = A ˆa , the scalar A is an algebraic number. It can be negative or positive. The direction of A will be the same as that of the unit vector ˆa if A > 0 and opposite to ˆa if A < 0. When we want to restrict to non-negative values, we use the symbol A and call it the modulus of A. Thus, A ³ 0 . Also note that though only the charge enclosed by the surface (ll ) was included above, the electric field E is due to the charge on the entire wire. Further, the assumption that the wire is infinitely long is crucial. Without this assumption, we cannot take E to be normal to the curved part of the cylindrical Gaussian surface. However, equation is approximately true for electric field around the central portions of a long wire, where the end effects may be ignored.
Note that when we write a vector A as a scalar multiplied by a unit vector, i.e., as A = A ˆa , the scalar A is an algebraic number. It can be negative or positive. The direction of A will be the same as that of the unit vector ˆa if A > 0 and opposite to ˆa if A < 0. When we want to restrict to non-negative values, we use the symbol A and call it the modulus of A. Thus, A ³ 0 . Also note that though only the charge enclosed by the surface (ll ) was included above, the electric field E is due to the charge on the entire wire. Further, the assumption that the wire is infinitely long is crucial. Without this assumption, we cannot take E to be normal to the curved part of the cylindrical Gaussian surface. However, equation is approximately true for electric field around the central portions of a long wire, where the end effects may be ignored.
1.2 Field due to a uniformly charged infinite plane sheet
Let s be the uniform surface charge density of an infinite plane sheet. We take the x-axis normal to the given plane. By symmetry, the electric field will not depend on y and z coordinates and its direction at every point must be parallel to the x-direction. We can take the Gaussian surface to be a
rectangular parallelepiped of cross sectional area A, as shown. (A cylindrical surface will also do.) As
seen from the figure, only the two faces 1 and 2 will contribute to the flux; electric field lines are parallel to the other faces and they, therefore, do not contribute to the total flux. The unit vector normal to surface 1 is in –x direction while the unit vector normal to surface 2 is in the +x direction. Therefore, flux E.dS through both the surfaces are equal and add up. Therefore the net flux through the Gaussian surface is 2 EA. The charge enclosed by the closed surface is sA. Therefore by Gauss’s law,
where ˆn is a unit vector normal to the plane and going away from it. E is directed away from the plate if s is positive and toward the plate if s is negative. Note that the above application of the Gauss’ law has brought out an additional fact: E is independent of x also. For a finite large planar sheet, Eq. is approximately true in the middle regions of the planar sheet, away from the ends.rectangular parallelepiped of cross sectional area A, as shown. (A cylindrical surface will also do.) As
seen from the figure, only the two faces 1 and 2 will contribute to the flux; electric field lines are parallel to the other faces and they, therefore, do not contribute to the total flux. The unit vector normal to surface 1 is in –x direction while the unit vector normal to surface 2 is in the +x direction. Therefore, flux E.dS through both the surfaces are equal and add up. Therefore the net flux through the Gaussian surface is 2 EA. The charge enclosed by the closed surface is sA. Therefore by Gauss’s law,
1.3 Field due to a uniformly charged thin spherical shell
Let s be the uniform surface charge density of a thin spherical shell of radius R. The situation has obvious spherical symmetry. The field at any point P, outside or inside, can depend only on r (the radial distance from the centre of the shell to the point) and must be radial (i.e., along the radius vector).
(i) Field outside the shell: Consider a point P outside the shell with radius vector r. To calculate E at P, we take the Gaussian surface to be a sphere of radius r and with centre O, passing through P. All points on this sphere are equivalent
relative to the given charged configuration. (That is what we
mean by spherical symmetry.) The electric field at each point
of the Gaussian surface, therefore, has the same magnitude
E and is along the radius vector at each point. Thus, E and
DS at every point are parallel and the flux through each
element is E DS. Summing over all DS, the flux through the
Gaussian surface is E × 4 pie r^2. The charge enclosed is
s × 4 pie R^2. By Gauss’s law
where q = 4 pie R2 s is the total charge on the spherical shell. Vectorially,relative to the given charged configuration. (That is what we
mean by spherical symmetry.) The electric field at each point
of the Gaussian surface, therefore, has the same magnitude
E and is along the radius vector at each point. Thus, E and
DS at every point are parallel and the flux through each
element is E DS. Summing over all DS, the flux through the
Gaussian surface is E × 4 pie r^2. The charge enclosed is
s × 4 pie R^2. By Gauss’s law
The electric field is directed outward if q > 0 and inward if q < 0. This, however, is exactly the field produced by a charge q placed at the centre O. Thus for points outside the shell, the field due to a uniformly charged shell is as if the entire charge of the shell is concentrated at its centre.(ii) Field inside the shell: In figure, the point P is inside the shell. The Gaussian surface is again a sphere through P centred at O. The flux through the Gaussian surface, calculated as before, is
E × 4 pie r^2. However, in this case, the Gaussian surface encloses no charge. Gauss’s law then gives
that is, the field due to a uniformly charged thin shell is zero at all points inside the shell. This important result is a direct consequence of Gauss’s law which follows from Coulomb’s law. The experimental verification of this result confirms the 1/r^2 dependence in Coulomb’s law.

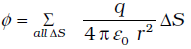






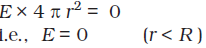





0 comments:
Post a Comment